Identifying Prime Polynomials in Algebra

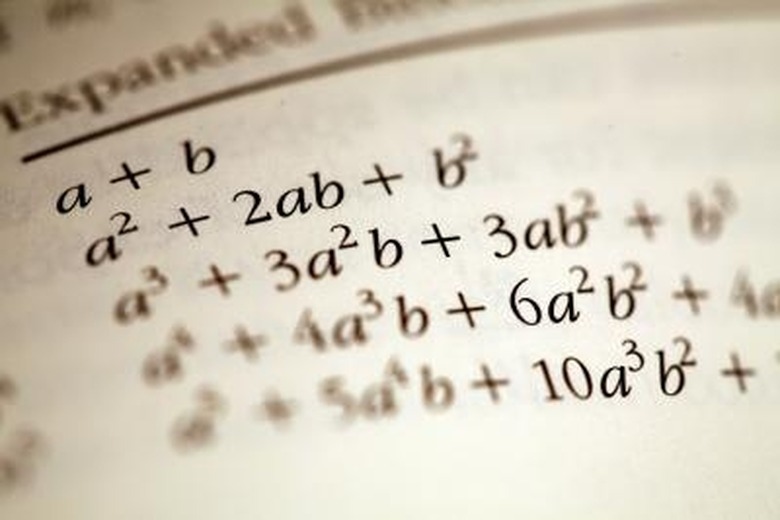
An algebra student gets stuck when exhausting all factoring steps, can’t find the answer, but forgets to consider a prime polynomial. These polynomials, like prime numbers, are in lowest common terms and you cannot factor them further. Here is how you recognize such an equation.

Step 1

Go through all the usual steps for factoring. First check for common monomial factors.

Step 2

Try the special formulas for factoring perfect squares, and then use the first formula for factoring a second-degree polynomial, x^2 + Bx + C, and see if it works.

Step 3

Apply the other special formula for factoring a second-degree polynomial of the form: Ax^2 + Bx + C.

Step 4

Exhaust all normal steps of factoring before deciding that you have a prime polynomial on your hands.

Step 5

Use the following as an example to help you learn to identify any prime polynomials you may come across: x^2 + 2x + 8. Set up a pair of two parentheses with the x’s in place: (x + )(x + )

Step 6

Look for two numbers whose product is 8 and sum is 2. Check for the 2 and 4 when both are either plus or both are minus, for 8. Try 1 and 8 with both plus or minus for the positive 8. None of these four sets of numbers equal 2.

Step 7

Declare the polynomial equation prime. You have looked at every possible way to factor the equation. It does not factor by a Greatest Common Factor or by special formulas. You have a prime polynomial on your hands.